Q1. What are the contributions mentioned in the paper "A cartesian grid embedded boundary method for the heat equation on irregular domains" ?
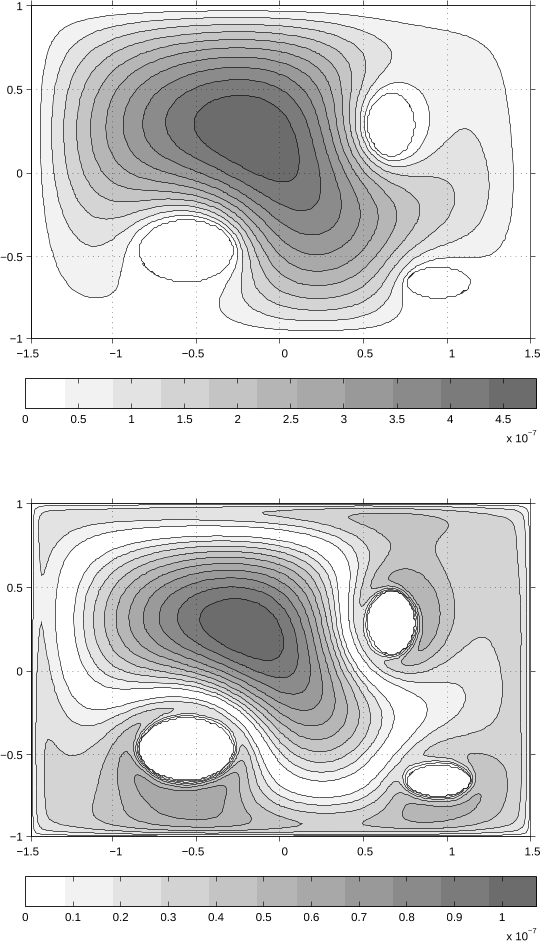
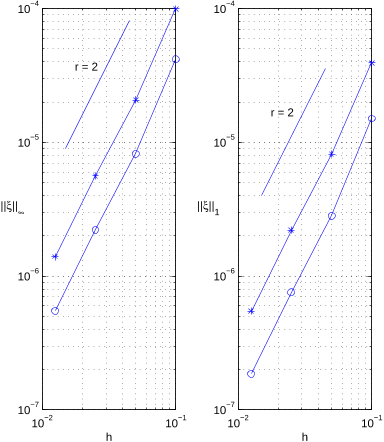
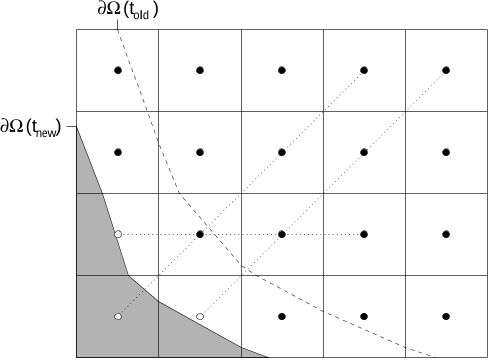
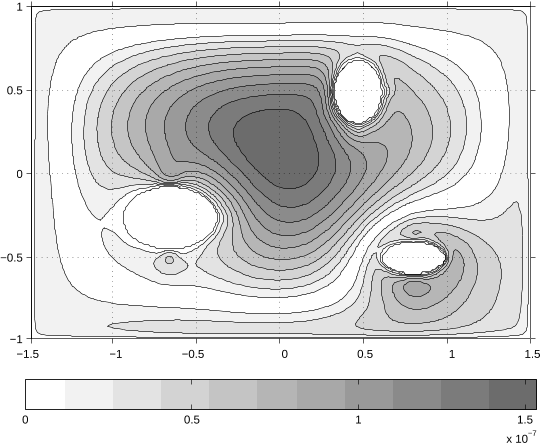
The authors present an algorithm for solving the heat equation on irregular time-dependent domains.
A Cartesian grid embedded boundary method for the heat equation on irregular domains
13 Nov 2001-Journal of Computational Physics (Academic Press Professional, Inc.)-Vol. 173, Iss: 2, pp 620-635
TL;DR: An algorithm for solving the heat equation on irregular time-dependent domains is presented, based on the Cartesian grid embedded boundary algorithm of Johansen and Colella, combined with a second-order accurate discretization of the time derivative.
About: This article is published in Journal of Computational Physics.The article was published on 2001-11-13 and is currently open access. It has received 161 citations till now. The article focuses on the topics: Mixed boundary condition & Boundary (topology).
Summary (1 min read)
1. INTRODUCTION
- For the time discretization, for the fixed-boundary problem the authors use either the Crank-Nicolson method or the method of Twizell, Gumel and Arigu (TGA) [10] .
- The authors algorithm is stable and achieves second-order accuracy both on problems with fixed domain and on problems with a time-dependent domain (t) with boundaries moving with constant velocities.
FIG. 1.
- Centers of cells in (t old ) are shown with solid circles, and centers of cells in (tnew) -(t old ) are shown with unfilled circles.
- The authors solve (19) numerically on a rectangular domain with three elliptically-shaped holes, with boundary conditions computed using the exact solution (18).
- In the moving-boundary problem, the holes move with constant velocities.
- With both fixed and moving boundaries, the authors solve two separate problems with different boundary conditions: Dirichlet conditions on all boundaries; Dirichlet conditions on the fixed external boundaries, but Neumann conditions on the boundaries of the ellipses.
Did you find this useful? Give us your feedback
Figures (15)
TABLE 3 
FIG. 6. Contour plots of absolute value of solution error to (19) at t = 1 for fixed boundaries, Dirichlet boundary conditions, h = 0:0125. Top figure is for Crank–Nicolson method, bottom figure for TGA method. 
FIG. 4. Solution error at t = 1 using Crank–Nicolson (stars) and TGA (circles), for the Dirichlet problem for (19) with fixed boundaries. Left-hand plot shows max norm, right-hand plot shows 1-norm. We see that both jj jj1 = O(h2) and jj jj1 = O(h2), indicating second-order accuracy. 
FIG. 5. Solution error at t = 1 using Crank–Nicolson (stars) and TGA (circles), for the Neumann problem for (19) with fixed boundaries. Left-hand plot shows max norm, right-hand plot shows 1-norm. We see that both jj jj1 = O(h2) and jj jj1 = O(h2), indicating second-order accuracy. 
FIG. 8. Contour plot of exact solution to (19) at t = 1 for moving-boundaryproblem. The dashed ellipses indicate the boundaries at t = 0. 
TABLE 1 
TABLE 2 Parameters for ellipses in (20), where = p 2=15 = 0:09428. 
FIG. 3. Contour plot of exact solution (18) to (19) at t = 1 with fixed boundaries. 
FIG. 10. Solution error at t = 1 in TGA method (circles) and Crank–Nicolson (stars), with Neumann conditions for (19) on moving boundaries. Left-hand plot shows max norm, right-hand plot shows 1-norm. 
FIG. 9. Solution error at t = 1 in TGA method (circles) and Crank–Nicolson method (stars), with Dirichlet conditions for (19) on moving boundaries. Left-hand plot shows max norm, right-hand plot shows 1-norm. 
FIG. 1. Centers of cells in (told) are shown with solid circles, and centers of cells in (tnew) - (told) are shown with unfilled circles. To estimate the value of U n at one of these latter points, we interpolate quadratically from values at the centers of three other cells in (told) forming a line with the new cell center. We pick whichever such line is closest in direction to the normal to the boundary at time t new. 
FIG. 2. From known values at points shown with solid circles on the moving boundary, we extrapolate to find values of (Dirichlet) or @ @n (Neumann) at points shown with unfilled circles, representing times told and tint on the boundary at time tnew. 
FIG. 11. Contour plot of absolute value of solution error to (19) at t = 1 for moving boundaries, Dirichlet boundary conditions, h = 0:0125 in TGA method. 
FIG. 12. Contour plot of absolute value of solution error to (19) at t = 1 for moving boundaries, Neumann boundary conditions, h = 0:0125 in TGA method. 
FIG. 7. Contour plots of absolute value of solution error to (19) at t = 1 for fixed boundaries, Neumann boundary conditions, h = 0:0125. Top figure is for Crank–Nicolson method, bottom figure for TGA method.
Citations
More filters
TL;DR: In this paper, the optimal local truncation error method (OLTEM) was extended to the 3D time-independent Helmholtz equation on irregular domains, where the stencil coefficients for the new approach are assumed to be unknown and are calculated by the minimization of the local truncations of the stochastic stencil equations.
Abstract: Here, we extend the optimal local truncation error method (OLTEM) recently developed in our papers to the 3D time-independent Helmholtz equation on irregular domains. Trivial unfitted Cartesian meshes and simple 27-point discrete stencil equations are used for 3D irregular domains. The stencil coefficients for the new approach are assumed to be unknown and are calculated by the minimization of the local truncation error of the stencil equations. This provides the optimal order of accuracy of the proposed technique. At similar 27-point stencils, the accuracy of OLTEM is two orders higher for the Dirichlet boundary conditions and one order higher for the Neumann boundary conditions compared to that for linear finite elements. The numerical results for irregular domains also show that at the same number of degrees of freedom, OLTEM is even much more accurate than high-order (up to the fifth order) finite elements with much wider stencils. Compared to linear finite elements with similar 27-point stencils, at accuracy of 0.1% OLTEM decreases the number of degrees of freedom by a factor of greater than 1000. This leads to a huge reduction in computation time. The new approach can be equally applied to the Helmholtz and screened Poisson equations.
1 citations
TL;DR: In this paper , the optimal local truncation error method (OLTEM) was developed for 2D time-dependent elasticity problems on irregular domains and trivial unfitted Cartesian meshes.
Abstract: The optimal local truncation error method (OLTEM) has been developed for 2‐D time‐dependent elasticity problems on irregular domains and trivial unfitted Cartesian meshes. Compact nine‐point uniform and nonuniform stencils (similar to those for linear finite elements on uniform meshes) are used with OLTEM. The stencil coefficients are assumed to be unknown and are calculated by the minimization of the local truncation error. It is shown that the second order of accuracy is the maximum possible accuracy for nine‐point stencils independent of the numerical technique used for their derivations. The special treatment of the Neumann boundary conditions has been developed that does not increase the size of the stencils. The cases of the nondiagonal and diagonal mass matrices are considered for OLTEM. The results of numerical examples are in agreement with the theoretical findings. They also show that due to the minimization of the local truncation error, OLTEM with the nondiagonal mass matrix is much more accurate than linear finite elements and than quadratic and cubic finite elements (up to the engineering accuracy of 0.1%–1%) at the same numbers of degrees of freedom. The proposed numerical technique can be efficiently used for many engineering applications including geomechanics.
1 citations
Journal Article•
TL;DR: This is the sixth self-assessment of the systems and services provided by the U.S. Department of Energy's National Energy Research Scientific Computing Center, describing many of the efforts of the NERSC staff to support advanced computing for scientific discovery.
Abstract: This is the sixth self-assessment of the systems and services provided by the U.S. Department of Energy's National Energy Research Scientific Computing Center, describing many of the efforts of the NERSC staff to support advanced computing for scientific discovery. The report is organized along the 10 goals set by our staff and outlines how we are working to meet those goals. Our staff applies experience and expertise to provide world-class systems and unparalleled services for NERSC users. At the same time, members of our organization are leading contributors to advancing the field of high-performance computing through conference presentations, published papers, collaborations with scientific researchers and through regular meetings with members of similar institutions. In the fast-moving realm of high-performance computing, adopting the latest technology while reliably delivering critical resources can be a challenge, but we believe that this self-assessment demonstrates that NERSC continues to excel on both counts.
1 citations
01 Jan 2010
TL;DR: A second-order interface tracking method: the Polygonal Area Mapping (PAM) method, which represents material areas explicitly as piecewise polygons and utilizes polygon-clipping algorithms from computational geometry, which is superior to existing VOF methods.
Abstract: The author presents a hybrid continuum-particle framework (HyPAM) consisting of three components. The first is a second-order interface tracking method: the Polygonal Area Mapping (PAM) method [Zhang and Liu 2008. J. Comput. Phys. 227(8):4063–4088], which represents material areas explicitly as piecewise polygons and utilizes polygon-clipping algorithms from computational geometry. In addition to its mesh-topology independence, the PAM method is free of numerical diffusion and tracks interfaces with or without singularities naturally and accurately. Both numerical experiments and theoretical analyses show that the PAM method is superior to existing VOF methods. The second component is a hybrid formulation for free-surface flows [Zhang and Liu 2009. J. Comput. Phys. 228(4):1312–1342]. A graph-based algorithm decomposes the water phase into a continuum zone and a particle zone, where the Euler equations and the free fall of rigid bodies are used as the governing equations, respectively and separately. The third component is the Explicit Jump Approximation (EJA) method for handling irregular solid boundaries in viscous flows [Zhang and Liu 2010. J. Comput. Phys. in press]. An analysis shows that the widely-used linear and quadratic ghost-cell approaches (GCA) are inconsistent and first-order accurate, respectively. As a remedy, the jump corrections at the solid-fluid interface are explicitly approximated and incorporated into the discretization of the Laplacian operator to obtain second-order convergence. Further developments of HyPAM include a fourth-order Navier-Stokes solver with adaptive mesh refinement (AMR), incorporating a particle method, and multi-material three-dimensional interface tracking.Copyright © 2010 by ASME
1 citations
Additional excerpts
...[23] showed the instability of C-N by a moving boundary calculation....
[...]
TL;DR: This method uses the non-traditional finite element method together with finite difference method to get solutions with second-order accuracy and is capable of dealing with matrix coefficient involving time, and the interfaces under consideration are sharp-edged interfaces instead of smooth interfaces.
Abstract: In this paper, we propose a numerical method for solving the heat equations with interfaces. This method uses the non-traditional finite element method together with finite difference method to get solutions with second-order accuracy. It is capable of dealing with matrix coefficient involving time, and the interfaces under consideration are sharp-edged interfaces instead of smooth interfaces. Modified Euler Method is employed to ensure the accuracy in time. More than 1.5th order accuracy is observed for solution with singularity (second derivative blows up) on the sharp-edged interface corner. Extensive numerical experiments illustrate the feasibility of the method.
1 citations
References
More filters
[...]
40,330 citations
Additional excerpts
...Similar approaches based on formally inconsistent discretizations at the irregular boundary have been used previously and observed to be stable [1, 9], so we expect that the extension to the more accurate boundary discretization should be straightforward....
[...]
Book•
07 Jan 2013
TL;DR: In this article, Leray-Schauder and Harnack this article considered the Dirichlet Problem for Poisson's Equation and showed that it is a special case of Divergence Form Operators.
Abstract: Chapter 1. Introduction Part I: Linear Equations Chapter 2. Laplace's Equation 2.1 The Mean Value Inequalities 2.2 Maximum and Minimum Principle 2.3 The Harnack Inequality 2.4 Green's Representation 2.5 The Poisson Integral 2.6 Convergence Theorems 2.7 Interior Estimates of Derivatives 2.8 The Dirichlet Problem the Method of Subharmonic Functions 2.9 Capacity Problems Chapter 3. The Classical Maximum Principle 3.1 The Weak Maximum Principle 3.2 The Strong Maximum Principle 3.3 Apriori Bounds 3.4 Gradient Estimates for Poisson's Equation 3.5 A Harnack Inequality 3.6 Operators in Divergence Form Notes Problems Chapter 4. Poisson's Equation and Newtonian Potential 4.1 Holder Continuity 4.2 The Dirichlet Problem for Poisson's Equation 4.3 Holder Estimates for the Second Derivatives 4.4 Estimates at the Boundary 4.5 Holder Estimates for the First Derivatives Notes Problems Chapter 5. Banach and Hilbert Spaces 5.1 The Contraction Mapping 5.2 The Method of Cintinuity 5.3 The Fredholm Alternative 5.4 Dual Spaces and Adjoints 5.5 Hilbert Spaces 5.6 The Projection Theorem 5.7 The Riesz Representation Theorem 5.8 The Lax-Milgram Theorem 5.9 The Fredholm Alternative in Hilbert Spaces 5.10 Weak Compactness Notes Problems Chapter 6. Classical Solutions the Schauder Approach 6.1 The Schauder Interior Estimates 6.2 Boundary and Global Estimates 6.3 The Dirichlet Problem 6.4 Interior and Boundary Regularity 6.5 An Alternative Approach 6.6 Non-Uniformly Elliptic Equations 6.7 Other Boundary Conditions the Obliue Derivative Problem 6.8 Appendix 1: Interpolation Inequalities 6.9 Appendix 2: Extension Lemmas Notes Problems Chapter 7. Sobolev Spaces 7.1 L^p spaces 7.2 Regularization and Approximation by Smooth Functions 7.3 Weak Derivatives 7.4 The Chain Rule 7.5 The W^(k,p) Spaces 7.6 DensityTheorems 7.7 Imbedding Theorems 7.8 Potential Estimates and Imbedding Theorems 7.9 The Morrey and John-Nirenberg Estimes 7.10 Compactness Results 7.11 Difference Quotients 7.12 Extension and Interpolation Notes Problems Chapter 8 Generalized Solutions and Regularity 8.1 The Weak Maximum Principle 8.2 Solvability of the Dirichlet Problem 8.3 Diferentiability of Weak Solutions 8.4 Global Regularity 8.5 Global Boundedness of Weak Solutions 8.6 Local Properties of Weak Solutions 8.7 The Strong Maximum Principle 8.8 The Harnack Inequality 8.9 Holder Continuity 8.10 Local Estimates at the Boundary 8.11 Holder Estimates for the First Derivatives 8.12 The Eigenvalue Problem Notes Problems Chapter 9. Strong Solutions 9.1 Maximum Princiles for Strong Solutions 9.2 L^p Estimates: Preliminary Analysis 9.3 The Marcinkiewicz Interpolation Theorem 9.4 The Calderon-Zygmund Inequality 9.5 L^p Estimates 9.6 The Dirichlet Problem 9.7 A Local Maximum Principle 9.8 Holder and Harnack Estimates 9.9 Local Estimates at the Boundary Notes Problems Part II: Quasilinear Equations Chapter 10. Maximum and Comparison Principles 10.1 The Comparison Principle 10.2 Maximum Principles 10.3 A Counterexample 10.4 Comparison Principles for Divergence Form Operators 10.5 Maximum Principles for Divergence Form Operators Notes Problems Chapter 11. Topological Fixed Point Theorems and Their Application 11.1 The Schauder Fixes Point Theorem 11.2 The Leray-Schauder Theorem: a Special Case 11.3 An Application 11.4 The Leray-Schauder Fixed Point Theorem 11.5 Variational Problems Notes Chapter 12. Equations in Two Variables 12.1 Quasiconformal Mappings 12.2 holder Gradient Estimates for Linear Equations 12.3 The Dirichlet Problem for Uniformly Elliptic Equations 12.4 Non-Uniformly Elliptic Equations Notes Problems Chapter 13. Holder Estimates for
18,443 citations
01 Jan 1997
TL;DR: In this paper, a class of partial differential equations that generalize and are represented by Laplace's equation was studied. And the authors used the notation D i u, D ij u for partial derivatives with respect to x i and x i, x j and the summation convention on repeated indices.
Abstract: We study in this chapter a class of partial differential equations that generalize and are to a large extent represented by Laplace’s equation. These are the elliptic partial differential equations of second order. A linear partial differential operator L defined by
$$ Lu{\text{: = }}{a_{ij}}\left( x \right){D_{ij}}u + {b_i}\left( x \right){D_i}u + c\left( x \right)u $$
is elliptic on Ω ⊂ ℝ n if the symmetric matrix [a ij ] is positive definite for each x ∈ Ω. We have used the notation D i u, D ij u for partial derivatives with respect to x i and x i , x j and the summation convention on repeated indices is used. A nonlinear operator Q,
$$ Q\left( u \right): = {a_{ij}}\left( {x,u,Du} \right){D_{ij}}u + b\left( {x,u,Du} \right) $$
[D u = (D 1 u, ..., D n u)], is elliptic on a subset of ℝ n × ℝ × ℝ n ] if [a ij (x, u, p)] is positive definite for all (x, u, p) in this set. Operators of this form are called quasilinear. In all of our examples the domain of the coefficients of the operator Q will be Ω × ℝ × ℝ n for Ω a domain in ℝ n . The function u will be in C 2(Ω) unless explicitly stated otherwise.
8,299 citations
"A Cartesian grid embedded boundary ..." refers background in this paper
...However, it is well known that, for any domain with smooth boundary, a smooth function can be extended to all of R with a bound on the relative increase in the C norms that depends only on the domain and (k; ) [5]....
[...]
TL;DR: In this paper, a second-order projection method for the Navier-Stokes equations is proposed, which uses a specialized higher-order Godunov method for differencing the nonlinear convective terms.
1,287 citations
"A Cartesian grid embedded boundary ..." refers methods in this paper
...The method described here, together with that in [6] for elliptic PDEs and [8] for hyperbolic PDEs, provide the fundamental components required for developing second-order accurate methods for a broad range of continuum mechanics problems in irregular geometries based on the predictor–corrector approach in [2]....
[...]
TL;DR: A numerical method for solving Poisson's equation, with variable coefficients and Dirichlet boundary conditions, on two-dimensional regions using a finite-volume discretization, which embeds the domain in a regular Cartesian grid.
470 citations
"A Cartesian grid embedded boundary ..." refers background or methods in this paper
...As in previous work on elliptic problems [6], our approach uses a finite-volume discretization, which embeds the domain in a regular Cartesian grid....
[...]
...We follow the approach described in [6, 7]....
[...]
...With Dirichlet boundary conditions as from (3), we compute an estimate of ∂ψ ∂n by interpolating from the grid values and the values at the boundaries; for details, see [6]....
[...]
...The method described here, together with that in [6] for elliptic PDEs and [8] for hyperbolic PDEs, provide the fundamental components required for developing second-order accurate methods for a broad range of continuum mechanics problems in irregular geometries based on the predictor–corrector approach in [2]....
[...]
...This is routine for the case in which the embedded boundary is contained in the finest level of refinement [6], but requires some additional discretization design when the embedded boundary crosses coarse–fine interfaces....
[...]