Q2. What are the main reasons why thin structures are increasingly used as structural components?
Thin structural systems composed of heterogeneous materials have been increasingly used as structural components particularly for impact, blast and crush applications, owing largely to their favorable impact resistance, energy absorption capability, specific strength and stiffness performance.
Q3. What is the effect of discretization of macroscopic and microscopic inelastic?
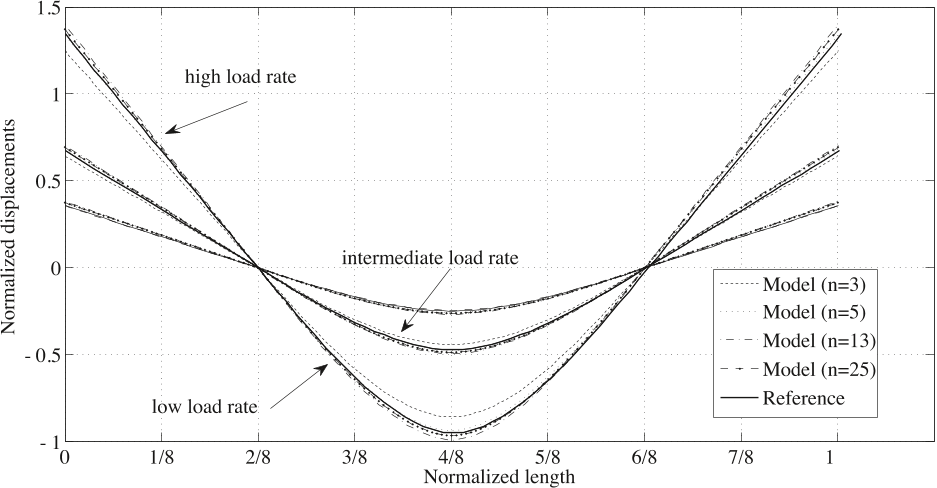
The discretization of macroscopic and microscopic inelastic strains results in reduction in number of kinematic equations for the system, which in turn improves the computational efficiency of the model.
Q4. What is the stiffness contrast between the matrix and reinforcement phases?
The stiffness contrast between the matrix and reinforcement phases is chosen to be EM/EF = 0.3, where, EM and EF are the Young’s Modulus of the matrix and fiber, respectively.
Q5. What is the eigendeformation concept used to evaluate the first order microscale problem?
For a fixed macroscopic state and time (i.e., evolution of the system is frozen), the eigendeformation concept may be invoked to evaluate the first order microscale problem.
Q6. What is the main problem with direct FEM modeling?
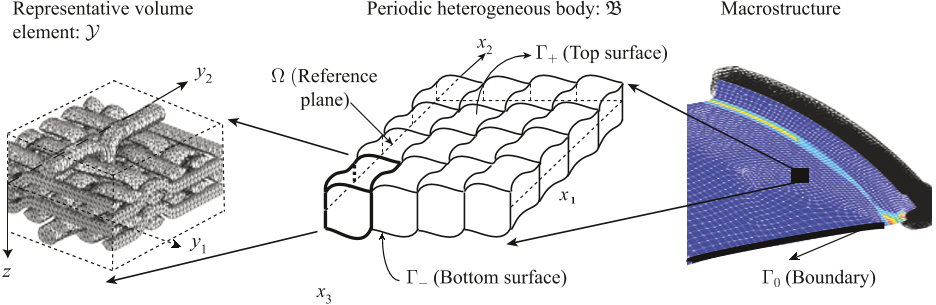
Despite reasonable accuracy and improved efficiency compared to direct finite element analysis using full resolution of the microstructure throughout the component scale, main difficulty with MHT-based structural models remains the high cost of solving 3-D microscopic boundary value problems on the representative volume element (RVE) domains to evaluate the macroscopic constitutive response.
Q7. What is the need for modeling and simulation tools for the prediction of failure?
There is a need for modeling and simulation tools capable of accurately representing the complex failure processes including matrix and fiber microcracking, interface debonding, delamination, fiber micro-buckling, kink banding and their interactions at the scale of the heterogeneities.
Q8. What is the eigenstrain based homogenization of the governing equations?
The eigenstrain based homogenization of the governing equations of a thin heterogeneous structure leads to a macroscopic problem with balance equations provided by Eqs. 43, 46 and 48 along with the constitutive relations (Eqs. 50 and 51).
Q9. What is the simplest way to determine the spatial derivative of f?
The spatial derivative of f ζ is calculated by the chain rule:f ζ ,i = δiα ( f,xα + 1 ζ f,yα ) + δi3 1 f,z (3)in which, a comma followed by an index denotes derivative with respect to the components of the position vector; a comma followed by a subscript variable xα or yi denotes a partial derivative with respect to the components of the macroscopic and microscopic position vectors, respectively; and δij denotes the components of the Kronecker delta.
Q10. How much damage has been observed in the failure load and displacements?
In case of fibers parallel to the loading direction a 166% and 140% increase have been observed in the failure load and displacements, respectively.
Q11. What is the current approach for thin heterogeneous plates?
The present approach is a generalization of the elastic theory proposed in Refs. [7, 8] for thin heterogeneous plates to account for the presence of inelastic and failure processes when subjected to static and dynamic loads.
Q12. Why are the damage evolution parameters chosen?
Damage evolution parameters are chosen to assure a linear dependence between the damage equivalent strain and evolution law (i.e., in Eq. 82, b(I) = 1).
Q13. How many plies are represented in the matrix?
The matrix, fiber tows in 0- and 90- directions and ply-interphase in each layer is represented by a single partition totaling 19 for 5 plies.
Q14. What are the eigenstrains for the macroscopic problem?
The damage induced inelastic strain tensors µ̄ij and µ̂ij account for the coupling between the microscopic and macroscopic problems.
Q15. What is the proposed approach for analyzing thin heterogeneous structures?
The proposed approach is computationally advantageous compared to direct nonlinear computational homogenization technique in two respects: (1) the necessity of evaluating nonlinear microscopic boundary value problems at all integration points in the macroscopic finite element mesh is eliminated using the eigendeformation concept, and; (2) necessity to resolve the thickness direction in the macroscopic scale is alleviated by considering a structural theory based approach.
Q16. What is the UGENS subroutine used to evaluate the macroscopic boundary value problem?
A commercial finite element software (Abaqus) is employed to evaluate the macroscopic boundary value problem summarized in Box 7.
Q17. What is the governing equation of failure of the heterogeneous body?
Failure of the heterogeneous body is considered as the progressive degradation of the material properties within the microconstituents when subjected to mechanical loads of sufficient amplitude.
Q18. What is the difference between the experimental and the simulated response?
The simulated response shows a nonlinear relationship in impact velocities close to the ballistic limit followed by a linearizing trend - similar to the experimental observations.