HAL Id: hal-00596639
https://hal.archives-ouvertes.fr/hal-00596639
Submitted on 30 May 2011
HAL is a multi-disciplinary open access
archive for the deposit and dissemination of sci-
entic research documents, whether they are pub-
lished or not. The documents may come from
teaching and research institutions in France or
abroad, or from public or private research centers.
L’archive ouverte pluridisciplinaire HAL, est
destinée au dépôt et à la diusion de documents
scientiques de niveau recherche, publiés ou non,
émanant des établissements d’enseignement et de
recherche français ou étrangers, des laboratoires
publics ou privés.
Breather mode in the many-electron dynamics of
semiconductor quantum wells
F. Haas, Giovanni Manfredi, P.K. Shukla, Paul-Antoine Hervieux
To cite this version:
F. Haas, Giovanni Manfredi, P.K. Shukla, Paul-Antoine Hervieux. Breather mode in the many-
electron dynamics of semiconductor quantum wells. Physical Review B: Condensed Matter and
Materials Physics (1998-2015), American Physical Society, 2009, 80 (7), pp.073301-1-073301-4.
�10.1103/PhysRevB.80.073301�. �hal-00596639�

Breather mode in the many-electron dynamics of semiconductor quantum wells
F. Haas,
1
G. Manfredi,
2
P. K. Shukla,
1
and P.-A. Hervieux
2
1
Institut für Theoretische Physik IV, Ruhr-Universität Bochum, D-44780 Bochum, Germany
2
Institut de Physique et Chimie des Matériaux de Strasbourg, BP 43, F-67034 Strasbourg, France
共Received 22 July 2009; published 14 August 2009
兲
We demonstrate the existence of a breather mode in the self-consistent electron dynamics of a semiconductor
quantum well. A nonperturbative variational method based on quantum hydrodynamics is used to determine the
salient features of the electron breather mode. Numerical simulations of the time-dependent Wigner-Poisson or
Hartree equations are shown to be in excellent agreement with our analytical results. For asymmetric quantum
wells, a signature of the breather mode is observed in the dipole response, which can be detected by standard
optical means.
DOI: 10.1103/PhysRevB.80.073301 PACS number共s兲: 73.63.Hs, 73.43.Lp, 78.67.De
I. INTRODUCTION
The many-electron dynamics in nanoscale semiconductor
devices, such as quantum wells and quantum dots, has re-
cently attracted a great deal of interest, mainly in view of
possible applications to the growing field of quantum
computing.
1
Particular attention has been devoted to inter-
subband transitions, which involve excitation frequencies of
the order of the terahertz.
2
On this time scale, various collec-
tive electronic modes can be excited. For instance, the elec-
tric dipole response is dominated by a strong resonance at
the effective plasmon frequency. This resonance 共known as
the Kohn mode兲
3
is characterized by rigid oscillations of the
electron gas, which, for perfectly parabolic confinement, are
decoupled from the internal degrees of freedom.
In this Brief Report, we show the existence of a new
distinct resonance—a monopole or “breather” mode—which
corresponds to coherent oscillations of the size of the elec-
tron gas around a self-consistent equilibrium. Breather
modes have been described in many areas of physics, such as
nuclear matter
4,5
共where they are known as giant monopole
resonances兲 and ultracold atom dynamics.
6,7
In experiments
on metallic nanoparticles, monopole oscillations of the ionic
structure have been observed, which manifest themselves as
slow modulations of the surface plasmon.
8
However, to the
best of our knowledge, previous investigations have not ad-
dressed the features of the breather mode in the self-
consistent dynamics of a confined electron gas. Although
quantum wells constitute a typical instance of such confined
systems, the present approach should equally apply to metal
nanoparticles and carbon-based systems such as fullerenes.
II. MODEL
Because of the translational symmetry in the transverse
plane, the problem reduces to a one-dimensional 共1D兲 one in
the x direction.
9,10
To model the electron dynamics, we use a
self-consistent quantum hydrodynamic model 共QHM兲 that
was originally derived for quantum plasmas
11,12
and metallic
nanostructures.
13
In the QHM, the evolution of the electron
density n共x ,t兲 and mean velocity u共x , t兲 is governed by the
continuity and momentum equations
n
t
+
x
共nu兲 =0, 共1兲
u
t
+ u
u
x
=−
1
m
ⴱ
n
P
x
−
1
m
ⴱ
V
eff
x
+
ប
2
2m
ⴱ
2
x
冉
x
2
冑
n
冑
n
冊
, 共2兲
where m
ⴱ
is the effective electron mass, ប is the reduced
Planck constant, P共x,t兲 is the electron pressure, and V
eff
=V
conf
共x兲+ V
H
共x , t兲 is the effective potential, which is com-
posed of a confining and a Hartree term. The Hartree poten-
tial obeys the Poisson equation, namely V
H
⬙
=−e
2
n/ , where e
is the magnitude of the electron charge and is the effective
dielectric permeability of the material. The term proportional
to ប
2
on the right-hand side of Eq. 共2兲 represents the quantum
force due to the so-called Bohm potential.
14
The above QHM can be derived from the self-consistent
Hartree equations
12
— or equivalently from the phase-space
Wigner-Poisson equations
15
—in the limit of long wave-
lengths compared to the Thomas-Fermi screening length. For
the sake of simplicity, we shall neglect exchange/correlation
corrections and assume Boltzmann statistics, which is a rea-
sonable approximation at moderate electron temperatures
T.
9,16
We also stress that the 1D model relies on the separa-
tion of the transverse and longitudinal directions, which may
be broken by collisional effects. However, such effects
should not be dominant on the fast time scales considered
here.
17
The pressure P共x,t兲 in Eq. 共2兲 must be related to the elec-
tron density n via an equation of state 共EOS兲 in order to close
our system of electron fluid equations. We take a polytropic
relation P = n
¯
k
B
T共n/ n
¯
兲
␥
, where k
B
is the Boltzmann constant,
␥
=3 is the 1D polytropic exponent, and n
¯
is a mean electron
density. For a homogeneous system 共where n
¯
=n
0
兲, this EOS
correctly reproduces the Bohm-Gross dispersion relation
12
in
quantum plasmas. For the inhomogeneous electron gas con-
sidered here, the choice of n
¯
is subtler and will be discussed
later.
We assume a parabolic confinement, with V
conf
=
1
2
0
2
m
ⴱ
x
2
, where the frequency
0
can be related to a ficti-
tious homogeneous positive charge of density n
0
via the re-
lation
0
=共e
2
n
0
/ m
ⴱ
⑀
兲
1/2
. We then normalize time to
0
−1
;
space to L
0
=共k
B
T/ m
ⴱ
兲
1/2
/
0
; velocity to L
0
0
; energy to
PHYSICAL REVIEW B 80, 073301 共2009兲
1098-0121/2009/80共7兲/073301共4兲 ©2009 The American Physical Society073301-1

k
B
T; and the electron number density to n
0
. Quantum effects
are measured by the dimensionless parameter H = ប
0
/ k
B
T.
We shall use typical parameters that are representative of
semiconductor quantum wells:
10
the effective electron mass
and the effective dielectric permeability are, respectively,
m
ⴱ
=0.067 m
e
and =13
0
, the equilibrium density is n
0
=4.7 ⫻10
22
m
−3
, and the filling fraction n
¯
/ n
0
=0.5. These
values yield an effective plasmon energy ប
0
=8.62 meV, a
characteristic length L
0
=16.2 nm, a Fermi temperature T
F
=51.8 K, and a typical time scale
0
−1
=76 fs. An electron
temperature T= 200 K then corresponds to a value H=0.5.
III. LAGRANGIAN APPROACH
In order to derive a closed system of differential equations
describing the breather mode, we fist express the quantum
hydrodynamical equations in a Lagrangian formalism. We
stress that this approach is not based on a perturbative ex-
pansion, and thus is not restricted to the linear regime. The
Lagrangian density corresponding to the system of Eqs. 共1兲
and 共2兲 reads as 共normalized units are used from now on兲
L =
1
2
冉
V
H
x
冊
2
− nV
H
− n
t
−
冕
n
W共n
⬘
兲dn
⬘
−
1
2
冉
n
冋
x
册
2
+
H
2
4n
冋
n
x
册
2
冊
− nV
conf
, 共3兲
where the independent fields are taken to be n,
, and V
H
.
The velocity field follows from the auxiliary function
共x , t兲
through u =
/
x. The quantity W共n兲 in Eq. 共3兲 originates
from the pressure, W ⬅兰
n
dP
dn
⬘
dn
⬘
n
⬘
=共3/ 2兲共n/ n
¯
兲
2
. Taking the
variational derivatives of the action S =兰Ldxdt with respect
to n,
, and V
H
, we obtain the Eqs. 共1兲 and 共2兲, as well as the
Poisson equation for V
H
.
The existence of a pertinent variational formalism can be
used to derive approximate solutions via the time-dependent
Rayleigh-Ritz trial-function method.
18
For this purpose, we
assume the electron density to have a
GAUSSIAN profile
n共x,t兲 =
A
exp
冋
−
共x − d兲
2
2
2
册
, 共4兲
where d共t兲 and
共t兲 are time-dependent functions that repre-
sent the center-of-mass 共dipole兲 and the spatial dispersion of
the electron gas, respectively. The constant A =N/
冑
2
,is
related to the total number of electrons in the well, N
=兰ndx. The above Ansatz is a natural one, because for a
negligible Hartree energy, V
eff
reduces to a harmonic oscilla-
tor potential.
The other fields to be inserted in the action functional are
and V
H
. The natural way to choose them is by requiring
that the continuity and Poisson equations are automatically
satisfied. The continuity equation is solved with n given by
Eq. 共4兲 together with u= d
˙
+共
˙
/
兲
, which leads to
=共
˙
/ 2
兲
2
+d
˙
, where
⬅x − d. An irrelevant gauge func-
tion was discarded in the calculation of
. The solution of the
Poisson equation with a
GAUSSIAN electron density is
V
H
=−A
e
−
2
/2
2
− A
冑
2
Erf
冉
冑
2
冊
+ const, 共5兲
where Erf is the error function. The integration constant is
chosen so that V
H
共⫾a兲= 0, with 2a being the total size of the
system, and letting a → ⬁ at the end of the calculation. As the
potential V
H
is not bounded, a divergence appears in the
Lagrangian density when integrated over space. However,
the divergent term does not depend on the dynamical vari-
ables d and
, so that it can be ignored.
Using the above Ansatz, one obtains the Lagrangian
L ⬅
1
冑
2
A
冕
Ldx =
d
˙
2
+
˙
2
2
−
d
2
+
2
2
+
冑
2
2
A
−
冑
3A
2
6n
¯
2
2
−
H
2
8
2
, 共6兲
which only depends on two degrees of freedom, namely the
dipole d and the variance
. The Euler-Lagrange equations
corresponding to the Lagrangian L read as
d
¨
+ d =0 共7兲
¨
+
=
冑
2A
2
+
冑
3A
2
3n
¯
2
3
+
H
2
4
3
. 共8兲
The quantum-well potential V
conf
manifests itself in the
harmonic forces on the left-hand side of both Eqs. 共7兲 and
共8兲. As expected, the equations for d and
decouple for
purely harmonic confinement. Equation 共7兲 describes rigid
oscillations of the electron gas at the effective plasmonic
frequency, i.e., the Kohn mode.
3,19
Equation 共8兲 describes the
dynamics of the breather mode, which features coherent os-
cillations of the width of the electron density. The three
terms in the right-hand side of Eq. 共8兲 correspond to the
Coulomb repulsion 共Hartree term兲, the electron pressure, and
the quantum Bohm potential, respectively. The breather Eq.
共8兲 can be written as
¨
=−dU / d
, where U共
兲 is a pseudo-
potential defined as U=
2
/ 2−
冑
2A
/ 2+
冑
3A
2
/ 共6n
¯
2
2
兲
+H
2
/ 共8
2
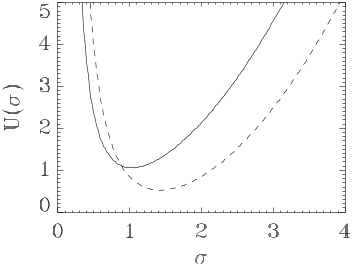
兲. From the shape of the pseudopotential 共Fig. 1兲,it
FIG. 1. Profiles of the pseudopotential U共
兲, for H = 0.5, A =0
共solid line兲 and H = 0.5, A =1 共dashed line兲. The fixed points are
0
=1.03 共A=0兲 and
0
=1.43 共A=1兲.
BRIEF REPORTS PHYSICAL REVIEW B 80, 073301 共2009兲
073301-2

follows that
will always execute nonlinear oscillations
around a stable fixed point
0
共A , H兲, which is a solution of
the algebraic equation U
⬘
共
0
兲=0.
So far, we have not specified the value of the average
density n
¯
that appears in the EOS, P = n
3
/ n
¯
2
, written in
normalized units. It is natural to assume that n
¯
takes some
value smaller than the peak density at equilibrium A /
0
. The
correct way to compute this value is to average the square of
the density using n itself, i.e., n
¯
2
⬅具n
2
典= 兰n
3
dx/ 兰ndx
=A
2
/ 共
冑
3
0
2
兲. A useful check can be performed by plugging
this expression into Eq. 共8兲 and neglecting the Hartree poten-
tial, which yields the equilibrium variance
0
=关1+共1
+H
2
兲
1/2
兴
1/2
/
冑
2. This expression displays the correct low- and
high-temperature limits for the quantum harmonic oscillator:
0
→ 1, for H→ 0; and
0
⬃
冑
H/ 2, for H Ⰷ 1.
With the above prescription for n
¯
, the pseudopotential be-
comes U =
2
/ 2−
冑
2A
/ 2+
0
2
/ 共2
2
兲+ H
2
/ 共8
2
兲. The fre-
quency ⍀ = ⍀共A , H兲 of the breather mode, corresponding to
the oscillations of
, can be obtained by linearizing the equa-
tion of motion 关Eq. 共8兲兴 in the vicinity of the stable fixed
point of U共
兲. The dependence of the breather frequency
with A 共i.e., with the electron density兲 is shown in Fig. 2. For
A=0 共i.e., without the Coulomb interaction兲 the exact fre-
quency is ⍀ =2
0
. For finite A, the breather frequency de-
creases and approaches ⍀ =
0
, for A→ ⬁. The latter limit
can be understood by noting that for large A the electron
density becomes flatter and flatter, due to the strong Cou-
lomb repulsion. Thus, in the limit A → ⬁ we end up with a
uniform electron density exactly neutralized by the ion den-
sity background. For such a homogeneous system, the
Bohm-Gross dispersion relation holds, which for long wave-
lengths yields ⍀=
0
. Indeed, if one computes the average
density using the prescription used for the EOS, one obtains
具n典= 兰n
2
dx/ 兰ndx= A / 共
冑
2
0
兲→ 1, for A → ⬁ 共see the inset of
Fig. 2兲. Thus, as expected, for large Coulomb effects the
average electron density becomes equal to the ion back-
ground density.
IV. SIMULATIONS
In order to check the validity of the above results, we
performed numerical simulations of the Wigner-Poisson
共WP兲 system, which is equivalent to the time-dependent Har-
tree equations.
15
In the normalized variables, the Wigner
pseudoprobability distribution f共x,
v
,t兲 satisfies the evolution
equation
f
t
+
v
f
x
− i
冕
dx
⬘
d
v
⬘
2
H
2
␦
V
eff
e
i共
v
−
v
⬘
兲x
⬘
/H
f共x,
v
⬘
,t兲 =0, 共9兲
and is coupled to the Poisson equation. In Eq. 共9兲,
␦
V
eff
⬅V
eff
共x + x
⬘
/ 2,t兲− V
eff
共x − x
⬘
/ 2,t兲. It is important to note that
this is a microscopic quantum mean-field model, much more
general than the hydrodynamic model on which our La-
grangian theory was based.
The initial condition used in the simulations is a quantum
canonical distribution for the harmonic oscillator at finite
temperature,
20
where the spatial width
0
has been adjusted
to the value obtained from the Lagrangian approach to ac-
count for the Coulomb repulsion. This is very close, but not
quite identical, to an exact equilibrium of the WP equations,
so that the width of the electron density starts to oscillate. We
then compute the evolution of the dispersion 具x
2
典
1/2
=共兰fx
2
dxd
v
/ 兰fdxd
v
兲
1/2
and its frequency spectrum, which
generally shows a sharp peak at a dominant frequency.
The results of the WP simulations are plotted in Fig. 2
共squares兲 and agree very well with the theoretical curve
based on the Lagrangian approach. The agreement slightly
deteriorates for larger values of A, because the electron den-
sity deviates from the
GAUSSIAN profile due to strong Cou-
lomb repulsion. This is clearly visible in Fig. 3, where we
represent the evolved density profiles for two values of A.
For A= 1, the profile is still approximately
GAUSSIAN,
whereas for A = 3 an intricate internal structure has devel-
oped. Nevertheless, even in this case, the error on the fre-
quency is still just over 3%.
Table I shows that the breather frequency depends weakly
on the parameter H 共and hence on the electron temperature兲.
Our theoretical results are in good agreement with the simu-
lations, except for H= 1. For this case, the frequency spec-
trum is particularly broad, denoting a significant fragmenta-
tion of the resonance.
FIG. 2. The breather frequency ⍀ as a function of A, for H
=0.5. Solid line: analytical results from the Lagrangian method.
Squares: the Wigner-Poisson 共WP兲 simulations. The inset shows the
mean electron density 具n典=A /
冑
2
0
as a function of A.
FIG. 3. Solid lines: electron density profiles at
0
t= 150 from
the WP simulations for H=0.5 and two values of A. The dashed
lines represent
GAUSSIAN distributions with the same width and the
same area as the numerical curves.
BRIEF REPORTS PHYSICAL REVIEW B 80, 073301 共2009兲
073301-3

V. NONPARABOLIC WELLS
For a parabolic potential well, there is no coupling be-
tween the breather and dipole modes, which may render the
experimental detection of the breather mode by optical
means difficult to realize in practice.
It can be shown that nonparabolic corrections do not in-
troduce any linear coupling if the confining potential is sym-
metric. A more interesting situation arises for asymmetric
wells,
21
which we model by adding a small cubic term to the
confining potential, V
cub
共x兲= 共K/ 3兲x
3
. The equations of mo-
tion are then
d
¨
+ d =−K共
2
+ d
2
兲, 共10兲
¨
+
=
冑
2A
2
+
冑
3A
2
3n
¯
2
3
+
H
2
4
3
−2K
d. 共11兲
Linearizing Eqs. 共10兲 and 共11兲 around the stable fixed point
共d
0
,
0
兲, we indeed find a coupling between the breather and
the dipole, with resonant frequencies ⍀
⫾
.
We model the coupling to the laser field by instanta-
neously shifting the initial dipole of a small quantity, i.e.,
d共0兲= d
0
+d
˜
. Figure 4 shows a typical spectrum obtained
from the numerical solution of Eqs. 共10兲 and 共11兲, which is
proportional to the optical absorption spectrum commonly
measured in the experiments. The sharp peaks correspond to
the resonant frequencies ⍀
−
=0.97 and ⍀
+
=1.58, which are
rather close to those obtained for parabolic confinement.
The breather mode can thus be triggered using a purely
dipolar excitation, and a clear signature of the breather fre-
quency can be observed in the optical absorption spectrum.
This opens the way to optically detecting the breather mode
by means of standard pump-probe experiments. Finally, the
methods used here could be readily extended to three-
dimensional nanostructures, and may find applications in re-
lated areas such as quantum free-electron lasers.
22
ACKNOWLEDGMENTS
This work was partially supported by the Alexander von
Humboldt Foundation and by the Agence Nationale de la
Recherche.
1
P. Zoller et al., Eur. Phys. J. D 36, 203 共2005兲.
2
J. N. Heyman et al., Appl. Phys. Lett. 72, 644 共1998兲.
3
W. Kohn, Phys. Rev. 123, 1242 共1961兲.
4
M. N. Harakeh, K. van der Borg, T. Ishimatsu, H. P. Morsch, A.
van der Woude, and F. E. Bertrand, Phys. Rev. Lett. 38, 676
共1977兲.
5
D. H. Youngblood, C. M. Rozsa, J. M. Moss, D. R. Brown, and
J. D. Bronson, Phys. Rev. Lett. 39, 1188 共1977兲.
6
K. Góral, M. Brewczyk, and K. Rzazewski, Phys. Rev. A 67,
025601 共2003兲.
7
Y. Zhou and G. Huang, Phys. Rev. A 75, 023611 共2007兲.
8
H. Portales et al., J. Chem. Phys. 115, 3444 共2001兲.
9
M. Santer, B. Mehlig, and M. Moseler, Phys. Rev. Lett. 89,
286801 共2002兲.
10
H. O. Wijewardane and C. A. Ullrich, Appl. Phys. Lett. 84, 3984
共2004兲.
11
G. Manfredi and F. Haas, Phys. Rev. B 64, 075316 共2001兲.
12
G. Manfredi, Fields Inst. Commun. 46, 263 共2005兲.
13
N. Crouseilles, P. A. Hervieux, and G. Manfredi, Phys. Rev. B
78, 155412 共2008兲.
14
C. L. Gardner and C. Ringhofer, Phys. Rev. E 53, 157 共1996兲.
15
G. Manfredi and P.-A. Hervieux, Appl. Phys. Lett. 91, 061108
共2007兲.
16
G. M. Gusev, A. A. Quivy, T. E. Lamas, J. R. Leite, A. K.
Bakarov, A. I. Toropov, O. Estibals, and J. C. Portal, Phys. Rev.
B 65, 205316 共2002兲.
17
J. N. Heyman, K. Unterrainer, K. Craig, B. Galdrikian, M. S.
Sherwin, K. Campman, P. F. Hopkins, and A. C. Gossard, Phys.
Rev. Lett. 74, 2682 共1995兲.
18
M. Matuszewski, E. Infeld, B. A. Malomed, and M. Trippen-
bach, Phys. Rev. Lett. 95, 050403 共2005兲.
19
J. F. Dobson, Phys. Rev. Lett. 73, 2244 共1994兲.
20
K. Imre et al., J. Math. Phys. 8, 1097 共1967兲.
21
E. Rosencher and P. Bois, Phys. Rev. B 44, 11315 共1991兲;E.
Dupont, et al., IEEE J. Quantum Electron. 42, 1157 共2006兲.
22
N. Piovella, M. M. Cola, L. Volpe, A. Schiavi, and R. Bonifacio,
Phys. Rev. Lett. 100, 044801 共2008兲.
TABLE I. The breather frequency ⍀ and the equilibrium width
0
for A= 1 and for various values of H.
H
0
⍀ 共theory兲 ⍀ 共sim.兲
0.00 1.41 1.58 1.60
0.50 1.43 1.59 1.60
1.00 1.47 1.60 1.51
1.50 1.52 1.61 1.57
2.00 1.59 1.63 1.63
3.00 1.73 1.66 1.70
FIG. 4. The frequency spectra of the dipole 共solid line兲 and
breather 共dashed兲 modes, for an asymmetric well with A =1, H
=0.5, and K =−0.1.
BRIEF REPORTS PHYSICAL REVIEW B 80, 073301 共2009兲
073301-4