




Did you find this useful? Give us your feedback




















122 citations
...Index Terms—Single-pair shortest path, KNN search, keyword search, road network, index, spatial databases Ç...
[...]
...3.3 G-tree Construction In this section, we present how to construct the G-tree....
[...]
58 citations
...Such queries, known as spatial keyword queries, which find the top-k objects of interest in terms of both spatial proximity and textual relevance to the query, have been extensively studied in recent years [6][13][15][20][21][25][26][27]....
[...]
...Technique Boolean keyword Continuous query Unknown path Static data objects Road network Safe region ROAD[13], G-tree[27], SP-tree[20], FBS[11] – – OA-kNN[21] – – YPK-CNN[24], CPM[16], GMA[17] CkNN[22] UNICONS[5] V∗-Diagram[18], MkSK[23], INS[14] LARC...
[...]
...SP-tree [20] deals with the problem of keyword search on large graphs by introducing a shortest path tree, thus the network distances between results and query are approximated by tree distances....
[...]
53 citations
...(2) Both methods [4, 26] assume that the index can reside in main memory....
[...]
...Given a graph G = (V,E) with vertex set V , and edge set E, the algorithm in [4] incurs a (2 log2 |V | − 1) approximation factor, which can be quite large given large values of |V |, and as shown in [26], the resulting error is significant in their empirical study in real graphs and good solutions can be missed....
[...]
...The authors of [26] point out that the error introduced by the star summary in [4] can be large....
[...]
...Both PMI and pivot-gs were implemented by the authors of [26]....
[...]
...As pointed out in [4] and [26], some keyword queries in a network are generated from a vertex inside the network with an interest of looking for vertices in a near-vicinity of the network....
[...]
40 citations
...The social distance is usually modeled as the shortest distance on the social graph [9], [10], [6], [5], [7]....
[...]
...(1) Social Relevance: The social distance for two vertices v ↔ v′ is adopted as the shortest distance [9], [10], [6], [5]....
[...]
22 citations
...[29] studied the top-k nearest keyword (k-NK) query over a graph....
[...]
...Therefore, the studied problems in [28], [29] are different from that in this paper, and their proposed techniques cannot be directed used for solving our problem in this paper....
[...]
...There are some works to study the variants of graph keyword search [28], [29]....
[...]
563 citations
...[23] is a seminal work on distance oracle that estimates distance with 2k − 1 stretch using an O(|V | 1 k ) sized index....
[...]
...[11] adapt the distance oracle [23] to answer 1-NK queries with 4k−5 stretch in O(k) time using an O(k|V | 1 k ) sized index....
[...]
545 citations
...The answer substructure can be a tree [12, 3, 13, 8, 10, 9], a subgraph [16, 17] or a r-clique [14]....
[...]
...v,13, [13, 13] g,2,[2,6] f,7,[7,8] r,1,[1,20]...
[...]
520 citations
...As we use a general graph model, existing k-NN solutions on spatial networks [15, 5, 6, 18, 19, 7] cannot be applied, as they usually rely on specialized structures that leverage properties of spatial data to optimize their solutions....
[...]
...Different from a large body of research on k-nearest neighbor (k-NN) search on spatial networks [15, 5, 6, 18, 19, 7], we define G as a general graph without coordinates....
[...]
...[15] uses network Voronoi polygons to divide a graph into disjointed subsets for kNN search....
[...]
...K nearest neighbor (k-NN) search has been extensively studied in spatial networks [15, 5, 6, 18, 19, 7]....
[...]
422 citations
357 citations
...The answer substructure can be a tree [12, 3, 13, 8, 10, 9], a subgraph [16, 17] or a r-clique [14]....
[...]
...Thus nodes with preorder in either of the two intervals [1, 1] and [7, 8] share the same entry edge (φ, r)....
[...]
...k Interval [1, 1] [2, 3] [4, 5] [6, 6] [7, 8]...
[...]
...By excluding the three intervals from [1, 20], two intervals [1, 1] and [7, 8] are left....
[...]
...v,13, [13, 13] g,2,[2,6] f,7,[7,8] r,1,[1,20]...
[...]
2With the tree distance formulation, the key operation in answering a k-NK query on a graph is to answer the k-NK query on a tree.
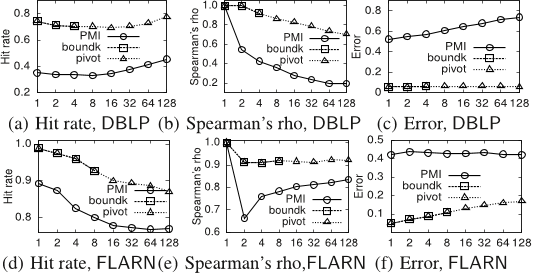
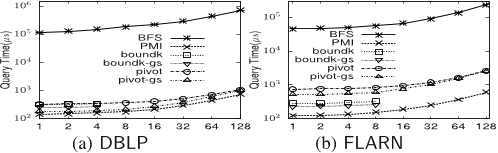
By keeping a global candidate list and removing duplicate index items, global storage reduces the index size of pivot by 61% on DBLP and 55% on FLARN.
Suppose the authors have an algorithm to compute RT on a tree T , the authors can solve the k-NK problem in a graph by merging RTi for each tree Ti, 1 ≤ i ≤ r.
As the authors transform a distance oracle on a graph into a set of shortest path trees, the original k-NK query on the graph can be reduced to answering the k-NK query on a set of trees.
Since CT(λ) keeps the structural information of all keyword nodes in T , it is sufficient to search only on CT(λ) to calculate candλ(v).
2THEOREM 5. Given a tree T (V, E), Algorithm 7 constructs a distance preserving balanced tree DT(T ) for T using O(|V | · log |V |) time and O(|V |) space.
This is because the complexity of pivot grows linearly with the tree depth,and the larger diameter of FLARN leads to a larger tree depth.
In order to reduce the average depth of nodes to optimize both index space and query processing time, the authors introduce a new structure called distance preserving balanced tree for T (V, E), denoted as DT(T ).
For each node v traversed, the authors merge candλ(v) into that of its parent node u by adding a distance dist(u, v) to the list candλ(v) (line 3-5).
Their second attempt is that, for each node v on the tree T (V, E) and each keyword λ, the authors precompute its k nearest nodes that contain λ.
Let (u, u′) = EEλ(q), since ENλ(q) is on the path from u to u′ on the tree T , the path from ENλ(q) to any keyword node in T will go through either u or u′.
Given a compact tree CT(λ) for a tree T and a keyword λ, the authors need to compute the candidate list candλ(v) for every node v on CT(λ).
For each pivot p of v as well as v itself, the authors calculate distT (p, v) on the original tree T , and add the element v : distT (p, v) to the candidate list candλ(p) (line 4-5).
The first traversal on CT(λ) is a bottom-up one, such that the candidate list on each node is propagated to all its ancestors on CT(λ).
Since a tree contains more structural information than a star, using tree distance will be more accurate than using witness distance for estimating the distance of two nodes.