
Edinburgh Research Explorer
Scaling of fracture systems in geological media
Citation for published version:
Bonnet, E, Bour, O, Odling, NE, Davy, P, Main, IG & Berkowitz, B 2001, 'Scaling of fracture systems in
geological media', Reviews of Geophysics, vol. 39, no. 3, pp. 347-383.
https://doi.org/10.1029/1999RG000074
Digital Object Identifier (DOI):
10.1029/1999RG000074
Link:
Link to publication record in Edinburgh Research Explorer
Document Version:
Publisher's PDF, also known as Version of record
Published In:
Reviews of Geophysics
Publisher Rights Statement:
Published in Reviews of Geophysics by the American Geophysical Union (2001)
General rights
Copyright for the publications made accessible via the Edinburgh Research Explorer is retained by the author(s)
and / or other copyright owners and it is a condition of accessing these publications that users recognise and
abide by the legal requirements associated with these rights.
Take down policy
The University of Edinburgh has made every reasonable effort to ensure that Edinburgh Research Explorer
content complies with UK legislation. If you believe that the public display of this file breaches copyright please
contact openaccess@ed.ac.uk providing details, and we will remove access to the work immediately and
investigate your claim.
Download date: 09. Aug. 2022

SCALING OF FRACTURE SYSTEMS IN GEOLOGICAL MEDIA
E. Bonnet, • O. Bour, 2 N. E. Odling, •'3 P. Davy, 2
I. Main, 4 P. Cowie, 4 and B. Berkowitz 5
Abstract. Scaling in fracture systems has become an
active field of research in the last 25 years motivated by
practical applications in hazardous waste disposal, hy-
drocarbon reservoir management, and earthquake haz-
ard assessment. Relevant publications are therefore
spread widely through the literature. Although it is rec-
ognized that some fracture systems are best described by
scale-limited laws (lognormal, exponential), it is now
recognized that power laws and fractal geometry provide
widely applicable descriptive tools for fracture system
characterization. A key argument for power law and
fractal scaling is the absence of characteristic length
scales in the fracture growth process. All power law and
fractal characteristics in nature must have upper and
lower bounds. This topic has been largely neglected, but
recent studies emphasize the importance of layering on
all scales in limiting the scaling characteristics of natural
fracture systems. The determination of power law expo-
nents and fractal dimensions from observations, al-
though outwardly simple, is problematic, and uncritical
use of analysis techniques has resulted in inaccurate and
even meaningless exponents. We review these tech-
niques and suggest guidelines for the accurate and ob-
jective estimation of exponents and fractal dimensions.
Syntheses of length, displacement, aperture power law
exponents, and fractal dimensions are found, after crit-
ical appraisal of published studies, to show a wide vari-
ation, frequently spanning the theoretically possible
range. Extrapolations from one dimension to two and
from two dimensions to three are found to be nontrivial,
and simple laws must be used with caution. Directions
for future research include improved techniques for
gathering data sets over great scale ranges and more
rigorous application of existing analysis methods. More
data are needed on joints and veins to illuminate the
differences between different fracture modes. The phys-
ical causes of power law scaling and variation in expo-
nents and fractal dimensions are still poorly understood.
1. INTRODUCTION
The study of fracture systems (terms in italic are
defined in the glossary, after the main text) has been an
active area of research for the last 25 years motivated to
a large extent by the siting of hazardous waste disposal
sites in crystalline rocks, by the problems of multiphase
flow in fractured hydrocarbon reservoirs, and by earth-
quake hazards and the possibility of prediction. Here we
define a fracture as any discontinuity within a rock mass
that developed as a response to stress. This comprises
primarily mode I and mode II fractures. In mode I
fracturing, fractures are in tensile or opening mode in
which displacements are normal to the discontinuity
walls (joints and many veins). Faults correspond to mode
•Nansen Center, Bergen, Norway.
2Geosciences Rennes, Universit6 Rennes, Rennes, France.
3Now at Rock Deformation Research Group, School of
Earth Sciences, University of Leeds, Leeds, England, United
Kingdom.
4Department of Geology and Geophysics, University of
Edinburgh, Edinburgh, Scotland, United Kingdom.
5Department of Environmental Sciences and Energy Re-
search, Weizmann Institute of Science, Rehovot, Israel.
II fractures, i.e., an in-plane shear mode, in which the
displacements are in the plane of the discontinuity. Frac-
tures exist on a wide range of scales from microns to
hundreds of kilometers, and it is known that throughout
this scale range they have a significant effect on pro-
cesses in the Earth's crust including fluid flow and rock
strength.
Early work was spread though a wide range of scales
from core through outcrop to aerial photographs and
satellite image scales. More recently, the manner in
which fracture system properties at different scales re-
late to each other, i.e., their scaling attributes, has re-
ceived increasing attention motivated by the promise of
statistical prediction that scaling laws offer. In earth-
quake hazard assessment, the main issue is the validity of
the Gutenberg-Richter law for predicting the probability
of occurrence of large earthquakes. In the case of the
hydrocarbon industry, such scaling laws provide a key to
predicting the nature of subseismic fracturing (below the
limit of seismic resolution), which can significantly influ-
ence reservoir and cap rock quality, from seismically
resolved faults. In groundwater applications, contami-
nant transport is particularly sensitive to the properties
and scaling of fracture systems. Fractal geometry is in
many cases well suited to the description of objects that
exhibit scaling behavior. The most important feature of
fractal geometry is the lack of any homogenization scale
Copyright 2001 by the American Geophysical Union.
8755-1209/01/1999 RG000074 $15.00
ß 347 ß
Reviews of Geophysics, 39, 3 / August 2001
pages 347-383
Paper number 1999RG000074
348 ß Bonnet et al.' SCALING OF FRACTURE SYSTEMS 39, 3 / REVIEWS OF GEOPHYSICS
or representative elementary volume. This has serious
consequences for the use of continuum mechanics for
describing the behavior of the lithosphere or the use of
equivalent porous media to describe the hydraulic be-
havior of fractured media, since both require the defi-
nition of a homogenization scale.
The numerous studies of fracture system scaling in
the literature do indeed suggest that such scaling laws
exist in nature. They also indicate, however, that such
scaling laws must be used with caution and with due
regard to the physical influences that govern their valid-
ity. Recent studies indicate that lithological layering
from the scale of a single bed to the whole crust is
reflected in fracture system properties and influences the
scale range over which individual scaling laws are valid.
The impact of these scaling laws for processes in the
Earth's crust such as fluid flow, rock strength, and seis-
mic hazard is a field that is now beginning to be explored
and promises to be an active area of research in the
future.
The subject of scaling in fracture systems has received
attention from workers in many fields including geology,
geophysics, physics, applied mathematics, and engineer-
ing. Communication between these different groups,
who often employ different terminologies, has not al-
ways been optimal. Thus we have included a tutorial that
attempts to define and make clear the links between the
different types of statistical description that appear in
the literature. The relevant literature is spread through-
out a wide variety of journals, and here we attempt to
pull together information from these different sources.
For the sake of brevity we have confined this review to
the scaling of fracture systems and have not included the
scaling properties of fracture surfaces themselves, for
which there is a large volume of literature. In the fol-
lowing, we have focused on the scaling properties of
fracture systems related either to their size distributions
or to their spatial properties. Fracture size is commonly
described by its length, by the tangential or perpendic-
ular displacement associated with the fracture, or by its
aperture, which is defined as the distance between the
fracture walls. We also outline the physical processes
that are responsible for scaling behavior and deal, in
some detail, with the practical problems of estimating
power law exponents and fractal dimensions.
2. STATISTICAL DESCRIPTION IN FRACTURE
CHARACTERIZATION
In recent years the power law distribution has been
increasingly employed to describe the frequency distri-
bution of fracture properties and geometry. However, a
power law is not an appropriate model in all cases, and
other distributions that have been used include the log-
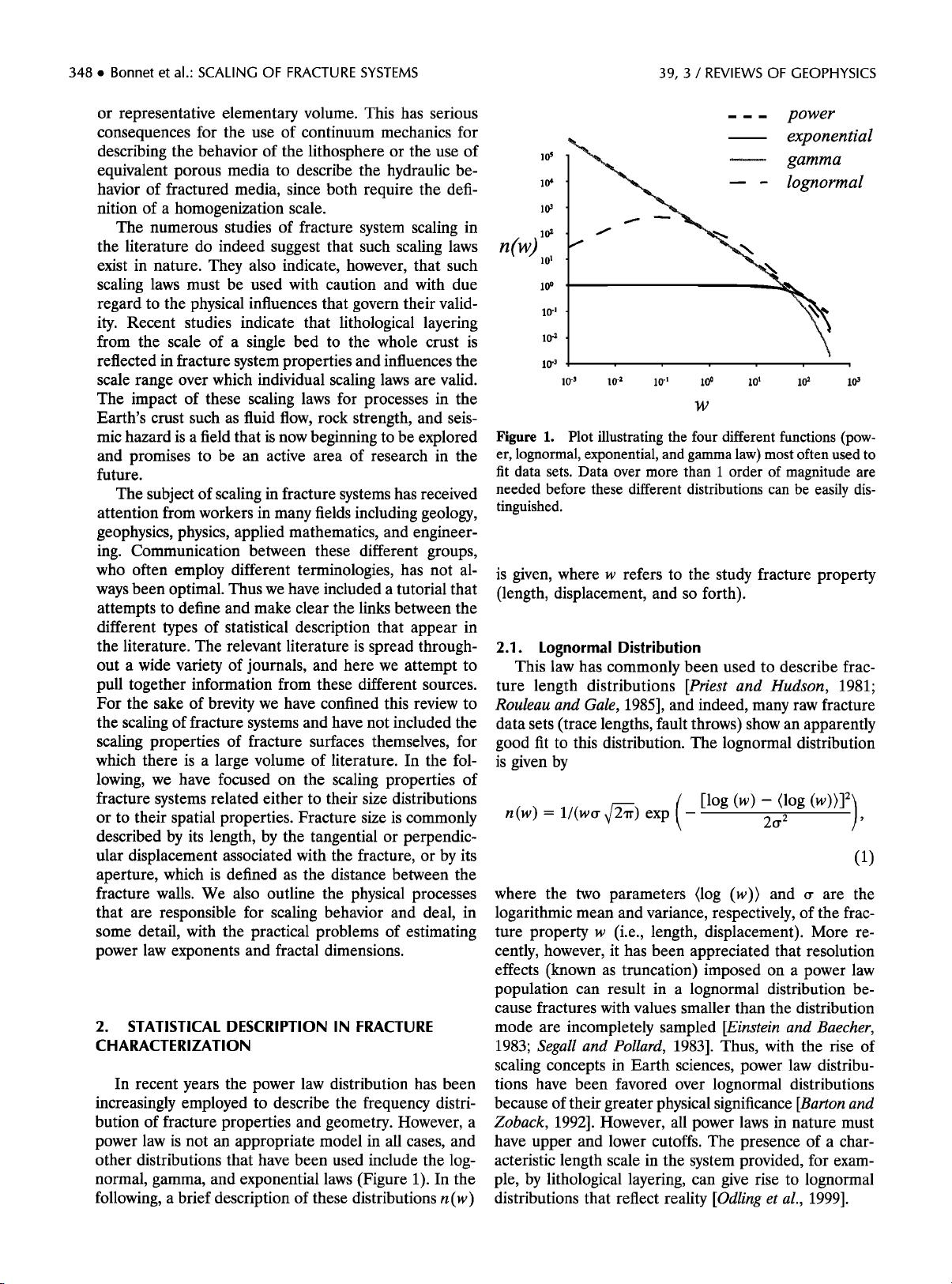
normal, gamma, and exponential laws (Figure 1). In the
following, a brief description of these distributions n(w)
lO t
10 0
10 a
10-a
10-3
power
exponential
gamma
lognormal
10 -3 10 2 10 4 10 0 10 • 10 •
w
Figure 1. Plot illustrating the four different functions (pow-
er, lognormal, exponential, and gamma law) most often used to
fit data sets. Data over more than 1 order of magnitude are
needed before these different distributions can be easily dis-
tinguished.
is given, where w refers to the study fracture property
(length, displacement, and so forth).
2.1. Lognormal Distribution
This law has commonly been used to describe frac-
ture length distributions [Priest and Hudson, 1981;
Rouleau and Gale, 1985], and indeed, many raw fracture
data sets (trace lengths, fault throws) show an apparently
good fit to this distribution. The lognormal distribution
is given by
[log (w) - (log (w))] 2)
n(w) = 1/(wcr x/2,r) exp - 2cr2 ,
where the two parameters (log (w)) and cr are the
logarithmic mean and variance, respectively, of the frac-
ture property w (i.e., length, displacement). More re-
cently, however, it has been appreciated that resolution
effects (known as truncation) imposed on a power law
population can result in a lognormal distribution be-
cause fractures with values smaller than the distribution
mode are incompletely sampled [Einstein and Baecher,
1983; Segall and Pollard, 1983]. Thus, with the rise of
scaling concepts in Earth sciences, power law distribu-
tions have been favored over lognormal distributions
because of their greater physical significance [Barton and
Zoback, 1992]. However, all power laws in nature must
have upper and lower cutoffs. The presence of a char-
acteristic length scale in the system provided, for exam-
ple, by lithological layering, can give rise to lognormal
distributions that reflect reality [Odling et al., 1999].

39, 3 / REVIEWS OF GEOPHYSICS Bonnet et al.: SCALING OF FRACTURE SYSTEMS ß 349
2.2. Exponential Law
This law has been used to describe the size of discon-
tinuities in continental rocks [Cruden, 1977; Hudson and
Priest, 1979, 1983; Priest and Hudson, 1981; Nur, 1982]
and in the vicinity of mid-oceanic ridges [Carbotte and
McDonald, 1994; Cowie et al., 1993b]. In these cases,
fracture growth results from a uniform stress distribu-
tion [Dershowitz and Einstein, 1988], and propagation of
fractures can be compared to a Poisson process [Cruden,
1977] resulting in an exponential distribution given by
n (w) = A 2 exp (-W/Wo), (2)
whereA 2 is a constant. The exponential law incorporates
a characteristic scale w0 (equation (2)) that reflects
either a physical length in the system, such as the thick-
ness of a sedimentary layer or the brittle crust [Cowie,
1998], or a spontaneous feedback processes during frac-
ture growth [Renshaw, 1999]. Numerical simulations
performed by Cowie et al. [1995] and experimental re-
sults of Bonnet [1997] have shown that exponential dis-
tributions of fracture length are also associated with the
early stages of deformation, when fracture nucleation
dominates over growth and coalescence processes.
An alternative to the power and exponential laws is
the stretched exponential that plays an intermediate role
[Laherrere and Sornette, 1998]. This law, which incorpo-
rates characteristic scales, can account for the observed
curvature in distributions and is related to large devia-
tions in multiplicative processes [Frisch and Sornette,
1997].
2.3. Gamma Law
The gamma distribution is a power law with an expo-
nential tail and is in common use in fault or earthquake
statistics and seismic hazard assessment [Davy, 1993;
Main, 1996; Kagan, 1997; Sornette and Sornette, 1999].
Any population that obeys this kind of distribution is
characterized by a power law exponent a and a charac-
teristic scale w0 (equation (3)).
It(W) =/t3 w-a exp (-W/Wo). (3)
In the physics of critical point phenomena [Yeomans,
1992, equation 2.12] the distribution of object size (i.e.,
length, displacement, aperture) or spacing may take this
form. The characteristic scale w0 may be related to (for
example) the correlation length in the spatial pattern,
where it implies an upper bound for fractal behavior
[Stauffer and Aharony, 1994], or may depend on defor-
mation rate [Main and Burton, 1984]. When w0 is greater
than the size of the system W max, the gamma law reduces
to a power law, and, conversely, a power law with a
strong finite size effect (see section 5.1.2) may also
resemble a gamma law.
2.4. Power Law
Numerous studies at various scales and in different
tectonic settings have shown that the distribution of
many fracture properties (i.e., length, displacement) of-
ten follows a power law (see sections 6 and 7):
It(W) = A4 w-a. (4)
Power law distributions have the important consequence
that they contain no characteristic length scale (equation
(4)). In nature the power laws have to be limited by
physical length scales that form the upper and lower
limits to the scale range over which they are valid. It is
now generally recognized that resolution and finite size
effects on a power law population can also result in
distributions that appear to be exponential or lognormal.
There appear to be physical grounds for why fracture
properties should follow power laws, and these are dis-
cussed in section 4. Since power law distributions are
playing an increasing role in our understanding of frac-
ture systems, the following sections concentrate largely
on this distribution and the estimation of its parameters.
3. DETERMINATION OF POWER LAW
EXPONENTS AND FRACTAL DIMENSIONS
FOR FRACTURE SYSTEMS: A TUTORIAL
There has been a tendency for workers from different
disciplines to use different methods for characterizing
power law fracture size distributions and fractal dimen-
sions. The value of the relevant power law exponent or
fractal dimension obtained depends on the method used,
which has led to some confusion in the literature. For
the benefit of those new to this field, the basic methods
of determining power law exponents from fracture pop-
ulation size data, and fractal dimensions from fracture
spatial data, are briefly reviewed here. Readers already
familiar with these methods may wish to skip to section
4.
3.1. Methods for Measuring Size Distributions
A power law may be assumed to be a reasonable
model for the size distribution of a fracture population
when the distribution trend on a log-log graph shows an
acceptable approximation to a straight line over a suffi-
cient scale range. Three different types of distribution
are commonly used to characterize fracture size data;
these are the frequency, frequency density, and cumula-
tive frequency distributions. In the literature, geologists
have most commonly used the cumulative distribution,
whereas geophysicists largely use the density distribution
because it is more amenable to integration for higher-
order moments. The value of the power law exponent
depends on the type of distribution on which the analysis
is based and also on bin type. Care must be taken to
compare like with like for scaling exponents quoted in
the literature. In this article we have chosen to use the
density distribution as the standard, since the other
forms may be easily derived from it. In this tutorial we

350 ß Bonnet et al.' SCALING OF FRACTURE SYSTEMS 39, 3 / REVIEWS OF GEOPHYSICS
TABLE 1. Relationship Between Distributions and Their
Exponents
Logarithmic Linear
Type of Distribution Bin Bin
Frequency a- 1 a
Density a a
Cumulative a- 1 a- 1
Comparison between the exponent values for the distributions
commonly used for the determination of power law length distribu-
tions.
have used fracture trace length l as an illustrative exam-
ple throughout.
For a population of fractures that follows a power
law, the manner in which the number of fractures de-
creases with size can be described by the frequency
distribution
N(l)- od-adl, (5)
where N(I ) is the number of fracture lengths that belong
to the interval [l, I + dl] for dl << l, o• is a density
constant, and a is the exponent. Where the bin size is
constant, the exponent equals a, but where the bin size
follows a logarithmic progression, the power law expo-
nent is a - 1, because d(ln (l)) = dl/l (see Table 1 and
Figure 2). This dependence of the exponent on the type
of bin is one reason why the density distribution expo-
nent, which is independent of the type of bin used, is
preferable. Another advantage of using the density dis-
tribution is the nature of the trend of the distribution at
large values where the number of elements belonging to
the interval can be very small (see section 5.1 for more
details). The density distribution n (l) corresponds to the
number of fractures N(l) belonging to an interval di-
vided by the bin size dl [Davy, 1993]:
l•l(l) = Od -a. (6)
As long as dl is small enough, the density distribution is
independent of the chosen bin size. The number of faults
N(l) gives the frequency distribution as in any standard
histogram plot. For a power law population, a log-log
plot of N(l) or n(l) versus I shows a straight line, the
slope of which gives the exponent of the power law
[Reches, 1986; Scholz and Cowie, 1990]. The choice of
the interval dl is critical in the sense that it defines the
degree of smoothing of the distribution trend, and a
small change in dl can lead to a significant change in the
number of fractures N belonging to each interval. Davy
[1993] has proposed an objective method for determin-
ing the size of interval at which n(l) shows the lowest
fluctuations.
The cumulative distribution represents the number of
fractures whose length is greater than a given length l
and corresponds to the integral of the density distribu-
tion n(l )
C(l) - n(l) dl, (7)
where /max is the greatest length encountered in the
network. Hence if n(l) is a power law characterized by
an exponent equal to a (equation (6)), the cumulative
distribution will be a power law for I << /max with an
Linear binning
N
10 3
10 2
1
10 ø
10 -I
[] Cumulative distribution C(l)=l-2
[] Frequency distribution N(l)=l-3
ß Density distribution n(l)=1-3
Logarithmic binning
N
10
10
10 ø
[] Cumulative distribution C(l)=l-2
[] Frequency distribution N(l)=l-2
ß Density distribution n(l)=1-3
I I I I I I I I I 10 4 • I • , , , , , I
100 10 100
Figure 2. Frequency, density, and cumulative distributions for theoretical population following a power law
with a density exponent of 3. Distributions have been calculated for (a) linear binning and (b) logarithmic
binning. The exponent changes according to the distribution and type of bin used.